Determing Continuity Removable and Non Removable

Removable Discontinuity

Removable discontinuity is a subtopic of the topic continuity (or continuous functions). A function that is not continuous is said to have a discontinuity. The graphs of such functions cannot be drawn without lifting a pencil. There are mainly two types of discontinuities: removable discontinuity and nonremovable discontinuity.

Let us learn more about removable discontinuity and why it is called so along with examples.

1.	What is Removable Discontinuity?
2.	Mathematical Definition of Removable Discontinuity
3.	How to Remove the Removable Discontinuity?
4.	Non Removable Discontinuity
5.	Difference Between Non Removable and Removable Discontinuities
6.	FAQs on Removable Discontinuity

What is Removable Discontinuity?

The removable discontinuity is a type of discontinuity of functions that occurs at a point where the graph of a function has a hole in it. This point does not fit into the graph and hence there is a hole (or removable discontinuity) at this point. Consider a function y = f(x) and assume that it has removable discontinuity at a point (a, f(a)). Then one of the following two things can happen in the graph of f(x):

Either f(a) is defined but (a, f(a)) doesn't lie on the curve of the function
or f(a) is NOT defined at all

We can see both the cases in the graph below:

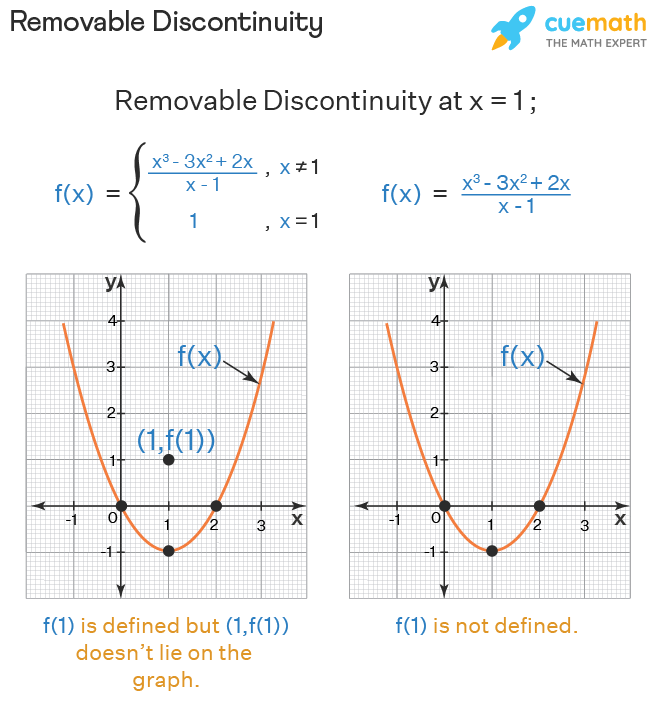
removable discontinuity

In the above figure, in both the graphs, it seems like the graph is entirely continuous except at the hole, and so redefining the function at that particular point (as f(1) = -1 in this case) makes the function continuous. The discontinuity can be easily removed this way, and hence the name removable discontinuity.

Mathematical Definition of Removable Discontinuity

Consider the first graph of the above figure. Clearly, the left-hand limit (lim ₓ → ₁₋ f(x)) and the right-hand limit (lim ₓ → ₁₊ f(x)) both are equal to -1. But f(1) = 1. i.e.,

lim ₓ → ₁₋ f(x) = lim ₓ → ₁₊ f(x) (= -1) but
The limits are NOT equal to f(1) (= 1)

In other words, lim ₓ → ₁ f(x) exists but is NOT equal to f(1) and we have seen that f(x) has a removable discontinuity at x = 1. Hence, we can define the removable discontinuity mathematically in one of the following ways:

A function f(x) is said to have a removable discontinuity at x = a if and only if limₓ → ₐ₋ f(x) = limₓ → ₐ₊ f(x) ≠ f(a).
[OR]
A function f(x) is said to have a removable discontinuity at x = a if and only if limₓ → ₐ f(x) ≠ f(a).

Let us prove the removable discontinuity in each of the graphs in the above figure.

The given function is f(x) = (x³ - 3x² + 2x) / (x - 1). We will compute its limit at x = 1.

lim ₓ → ₁ f(x) = lim ₓ → ₁ (x³ - 3x² + 2x) / (x - 1)
= lim ₓ → ₁ [ x (x² - 3x + 2) ] / (x - 1)
= lim ₓ → ₁ [ x (x - 1) (x - 2) ] / (x - 1)
= lim ₓ → ₁ [ x (x - 2) ] (as (x - 1) got canceled)
= 1 (1 - 2)
= -1

First Graph: f(1) = 1 and in this case, lim ₓ → ₁ f(x) ≠ f(1).

Second Graph: f(1) = (1³ - 3(1)² + 2(1)) / (1 - 1) = 0/0, which is an indeterminate form and hence f(1) is NOT defined. Here also, lim ₓ → ₁ f(x) ≠ f(1).

Thus, the removable discontinuities in both graphs are justified by the mathematical definition.

How to Remove the Removable Discontinuity?

In each of the above examples, there was a removable discontinuity at x = 1 and it happened just because lim ₓ → ₁ f(x) ≠ f(1). This can be easily removed simply by defining f(1) as lim ₓ → ₁ f(x). In the above example, if we set the function as follows, the function will be continuous at x = 1.

\(f(x)=\left\{\begin{array}{ll}
\frac{x^{3}-3 x^{2}+2 x}{x-1}, & \text { if } x \neq 1 \\
-1, & \text { if } x = 1
\end{array}\right.\)

As we found earlier, lim ₓ → ₁ f(x) = -1 and by the above definition of function, f(1) = -1. Hence, lim ₓ → ₁ f(x) = f(1) in this case, and hence the function is continuous at x = 1.

Thus, here are the steps to remove the removable discontinuity of a function f(x) at x = a.

Find limₓ → ₐ f(x) (call it as L).
Define f(a) = L.

Non Removable Discontinuity

In contrary to the removable discontinuity, a function f(x) has non removable discontinuity at x = a if the limit limₓ → ₐ f(x) does not exist. There are two types of nonremovable discontinuities:

Jump Discontinuity
Infinite Discontinuity

Jump Discontinuity

If a function f(x) has a jump discontinuity at x = a, then the curve of the function jumps at x = a from one place to another place. This is because the left-hand limit (limₓ → ₐ₋ f(x)) and the right-hand limit (limₓ → ₐ₊ f(x)) exist but they are NOT equal. Since the limit itself doesn't exist in jump discontinuity, we don't need to worry about whether limit is equal to f(a). The jump discontinuity looks as follows:

Jump discontinuity is a non removable discontinuity

Hence, the jump discontinuity of a function f(x) at x = a is defined mathematically as follows:

limₓ → ₐ₋ f(x) and limₓ → ₐ₊ f(x) exist and they are NOT equal
[OR]
limₓ → ₐ₋ f(x) ≠ limₓ → ₐ₊ f(x)

Infinite Discontinuity

If a function has an infinite discontinuity then one or both of the left-hand and right-hand limits is equal to ± ∞. For example, a function f(x) has infinite discontinuity when limₓ → ₐ₋ f(x) = ∞ and/or limₓ → ₐ₊ f(x) = -∞. The graph of a function having infinite discontinuity looks as follows:

Infinite discontinuity is a non removable discontinuity

By the above graph, it is so obvious that an infinite discontinuity occurs at a vertical asymptote. The above graph has a vertical asymptote at x = a.

Difference Between Non Removable and Removable Discontinuities

We now have a very clear idea of what is removable discontinuity and nonremovable discontinuity. The following table summarizes the differences between them. In each case, assume that a function f(x) is discontinuous at x = a.

Removable Discontinuity	Non Removable Discontinuity
1. Limit exists at the point of discontinuity.	1. Limit does not exist at the point of discontinuity.
2. limₓ → ₐ f(x) ≠ f(a)	2. When limₓ → ₐ f(x) doesn't exist, we don't need to think about whether the limit equals f(a).
3. Graphically: This occurs when the graph is such that it is possible to make it continuous just by filling the gap of discontinuity.	3. Graphically: This occurs when the graph jumps or tends to ± ∞ at x = a.
4. Mathematically: This mostly occurs when (x - a) is a factor of both numerator and denominator (or in other words, there is a hole at x = a)	4. Mathematically: This mostly occurs when left and right-hand limits are not the same (or) when x = a is a vertical asymptote.

Important Notes on Removable Discontinuity:

There are 3 types of discontinuities: (i) Removable discontinuity (ii) Jump discontinuity and (iii) Infinite discontinuity
Removable discontinuity formula: limₓ → ₐ f(x) ≠ f(a)
Jump discontinuity formula: limₓ → ₐ₋ f(x) ≠ limₓ → ₐ₊ f(x)
Infinite discontinuity formula: limₓ → ₐ₋ f(x) and/or limₓ → ₐ₊ f(x) = ∞ or -∞

☛ Related Topics:

Limits Calculator
Calculus Calculator
Derivatives

Removable Discontinuity Examples

Example 1: Prove that the function f(x) = sin x/x has a removable discontinuity at x = 0. Also, how can we remove the discontinuity here?

Solution:

The given function is, f(x) = sin x/x. We will compute its limit at x = 0.

limₓ → ₀ f(x) = limₓ → ₀ (sin x / x)
= limₓ → ₀ (cos x / 1) ( by L'Hopital's rule)
= (cos 0) / 1
= 1/1
= 1

But f(0) = (sin 0) / 0 = 0/0, which is not defined.

So limₓ → ₀ f(x) ≠ f(0). Hence f(x) has a removable discontinuity at x = 0 and it can be removed by defining the function as follows:

f(x) = \(\left\{\begin{array}{ll}
\dfrac{\sin x}{x}, & \text { if } x \neq 0 \\
1, & \text { if } x=0
\end{array}\right.\)

Answer: We proved that f(x) has a removable discontinuity at x = 0 and it can be removed by defining the function as above.
Example 2: Does the following function has a removable discontinuity or jump discontinuity at x = 1? Justify: f(x) = \(\left\{\begin{array}{ll}
x+2, & \text { if } x \leq 1 \\
x-2, & \text { if } x>1
\end{array}\right.\)

Solution:

Let us compute the left and right hand limits of f(x) at x = 1.

lim ₓ → ₁₋ f(x) = lim ₓ → ₁ x + 2 = 1 + 2 = 3

lim ₓ → ₁₊ f(x) = lim ₓ → ₁ x - 2 = 1 - 2 = -1

So lim ₓ → ₁₋ f(x) ≠ lim ₓ → ₁₊ f(x).

Answer: Thus, the given function has a jump discontinuity at x = 1.
Example 3: Does the following function has a removable discontinuity or nonremovable discontinuity at x = 1? If it is nonremovable, what type of discontinuity it is? f(x) = (x² + 2x + 1) / (x² - 2x + 1)

Solution:

The given function can be simplified as follows:

f(x) = (x² + 2x + 1) / (x² - 2x + 1)
= (x + 1)² / (x - 1)²

Oops! Nothing is getting canceled. So f(x) cannot have a hole (a removable discontinuity) at x = 1. Thus, f(x) has a nonremovable discontinuity at x = 1.

In particular, f(x) has a vertical asymptote at x = 1 and hence it has an infinite discontinuity at x = 1.

Answer: Nonremovable, in particular, infinite discontinuity.

go to slidego to slidego to slide

Have questions on basic mathematical concepts?

Become a problem-solving champ using logic, not rules. Learn the why behind math with our certified experts

Book a Free Trial Class

Practice Questions on Removable Discontinuity

go to slidego to slide

FAQs on Removable Discontinuity

What is a Removable Discontinuity Example?

A function y = f(x) has a removable discontinuity at x = a when limₓ → ₐ f(x) ≠ f(a). For example, f(x) = (x² - 9) / (x - 3). Then limₓ → ₃ f(x) = limₓ → ₃ [(x -3)(x+3)] / (x - 3) = limₓ → ₃ (x + 3) = 3 + 3 = 6. But f(3) = (3² - 9) / (3 - 3) = 0/0. So limₓ → ₃ ≠ f(3) and hence f(x) has a removable discontinuity at x = 3.

Does Every Piecewise Function have a Removable Discontinuity?

No, every piecewise function doesn't need to be discontinuous, and hence it doesn't need to have a removable discontinuity. For example: f(x) = {(x² - 9) / (x - 3), when x ≠ 3; and 6, when x = 3} is continuous everywhere (in particular it doesn't have any removable discontinuty at x = 3).

What is Jump Discontinuity?

The jump continuity, as its name suggests, occurs when the curve jumps from one place to the other place at the point where it has jump continuity. In other words, the left-hand-sided limit and right-handed-sided limits at the point with jump discontinuity are NOT equal.

How Do You Know if a Discontinuity is Removable?

If the limit of a function exists at a point (i.e., both left-hand and right-hand limits at that point exist, and also they are equal) but the limit is NOT equal to the value of the function at that point, then the discontinuity is called removable. We can remove that kind of discontinuity by redefining the value of the function to be the limit of the function.

What are Removable and Non Removable Discontinuities?

The graph of a function has a removable discontinuity at a point if there is just a hole at that point. On the contrary, the graph of a function has non removable discontinuity if either the graph jumps at that point or a part of the graph tends to ± ∞ at that point.

What is the Difference Between Removable and Jump Discontinuity?

A function f(x) has removable discontinuity at x = a if limₓ → ₐ f(x) exists but it is not equal to f(a). A function f(x) has jump discontinuity at x = a if limₓ → ₐ f(x) itself doesn't exist.

How many Types of Nonremovable Discontinuity are there?

There can be two types of non-removable discontinuity.

Jump discontinuity: In this, the LHL and RHL exist but are not equal.
Infinite discontinuity: In this, either LHL or RHL or both tend to ∞ or -∞.

What is the Difference Between Infinite and Removable Discontinuity?

Assume that a function f(x) has a discontinuity at a point x = a. Then:

the discontinuity is infinite if one/both of the left and right-hand limits are equal to ± ∞
the discontinuity is removable of both left and right-hand limits are equal and these limits are not equal to f(a).

wetherbeecamoul.blogspot.com

Source: https://www.cuemath.com/calculus/removable-discontinuity/